Talk on weakened higher dimensional enrichment:

In an enriched category A (over V) the role of composition is taken over by special morphisms in the monoidal category V. They appear as follows:

M:A(Y,Z)\otimes A(X,Y) --> A(X,Z).

I'll call a string of these hom-objects (such as the string of length two in the domain of M) composable if they can be reduced to a single hom-object by repeated uses of M. Of course the parenthization of the original string matters. Keep in mind then also the associator in V, /alpha, used to get from one parenthization to another. For a string of length n we can draw the associahedron K_n and put the various parenthizations at the vertexes, and the associators on the edges.

In my recent paper:

Vertically Iterated Classical Enrichment Also available from the arXiv and from TAC.

I define iterated enrichment in the sense that we enrich over V-Cat, the category of enriched categories over V, and etc. I would like to weaken this concept. The next slide reviews some of the paper and future plans.

The idea is that since, when enriching over V-n-Cat, composition morphisms

M_1:U(B,C)\otimes U(A,B) -> U(A,C)

are enriched n-functors, then the pentagon they are usually required to satisfy exactly can instead be filled in with an (invertible) enriched n-natural transformation M_2. Then we draw the pentagonal diagram of K_4 with vertices labeled by parenthesized strings of composable hom-objects, and compose each vertice by use of M_1 to a common final hom-object. What this does is to subdivide K_4 into two-cells that we fill in again with instances of M_2. We are now looking at a polyhedron with one of the pentagonal faces being K_4.

The next slide shows this process.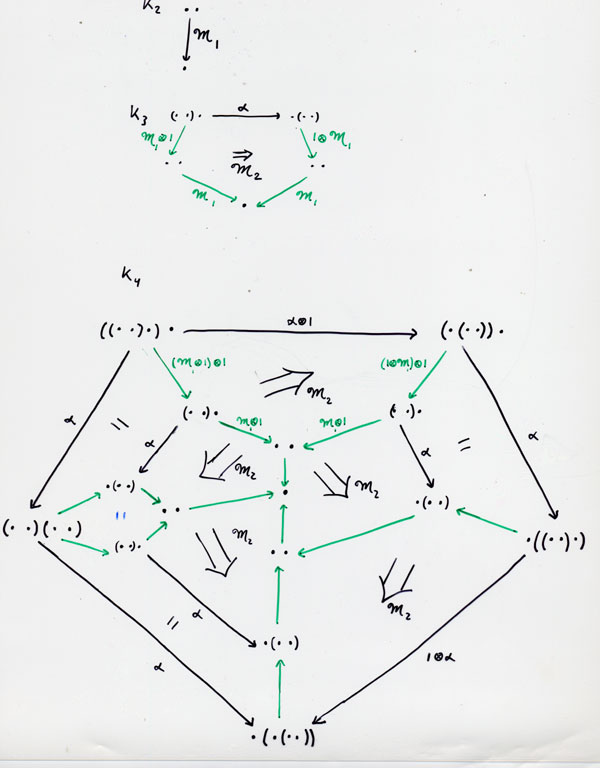

There are exactly two 2-dimensional paths that make up the front and back of the polyhedron. Between the latter there should now exist an enriched modification M_3. The next slide shows the same polyhedron as in the flattened picture at the end of the last slide, but drawn in 3 dimensions.

In general we continue subdividing associahedra with vertices labeled by composable hom-objects and filling in with a k-cell M_k until at last M_n+2 is an identity morphism--i.e. the last diagram made by composing the vertices of the last associahedron and filling in with M_n+1 is required to commute.

The last slide shows just the beginning of the next step: I've filled in one of the quadrilaterals on K_5 to show that eventually an identity 3-cell will fit nicely into the wedge with the quadrilateral as its base and the final hom-object as its point.

Further work: see slides and pdf of my talk to the workshop on n-categories at the IMA. These include discussion of weak enriched morphisms as well as an operad viewpoint. Higher Enrichment: N-Fold Operads and Enriched N-Categories, Delooping and Weakening

 

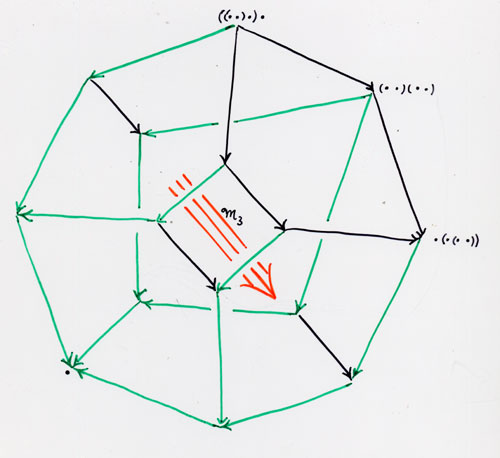
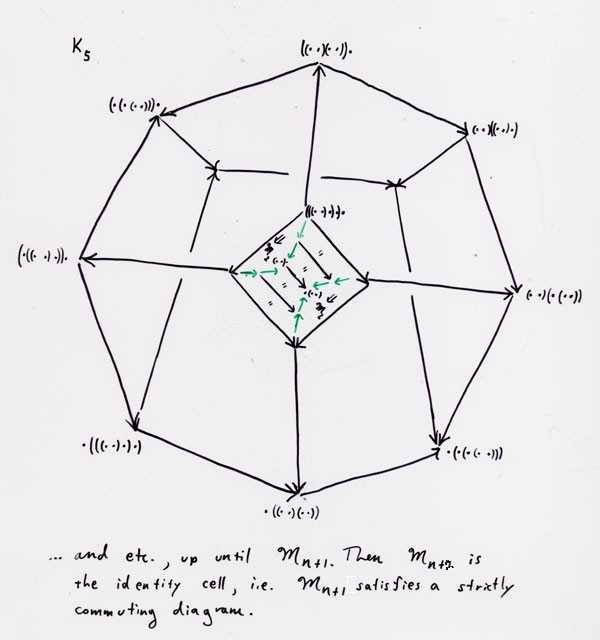
Back to research page.

Revision Date: