grope constrained braids

Grope constrained braids with associahedra indexing
Examples
Presentations
Filtrations
Intersections
Brunnian braids
Closing
Further investigation
There are famous connections between the derived and lower central series of the fundamental group of a topological space and grope inclusions into that space. The kth term in the derived series of the fundamental group of a space consists of the elements which bound a map from a grope of height k to the space. The kth term in the lower central series of the fundamental group of a space consists of the elements which bound a map from a grope of class k to the space. Braid groups of n strands arise as the fundamental groups of configuration spaces of n points in the two dimensional disk. There are also hints of a geometrical characterization of the derived and lower central series of the braid groups. The subgroup of the pure braid group made up of all pure braids such that all (pairwise) linking numbers of the closure of the braid are zero is the second lower central subgroup of the pure braid group. In general, for the n-strand pure braid group, the Brunnian braids coincide with the (n-1)-st lower central subgroup up to link homotopy. (communication from J. Hughes) We will discuss a family of filtrations of the braid groups that illuminates connections between the known facts. We consider braids on n strands for which we are also given a facet f of K(n), the Stasheff associahedron, which determines a parenthetical partition of the strands. The Stasheff associahedra are combinatorial polytopes whose vertices correspond to the complete partitions of a string of given length. Higher dimensional faces correspond to incomplete partitions which are compatible along shared lower dimensional faces. Grope annuli of type corresponding to a binary tree are CW complexes made up of cylinders of any genus but with punctured tori iteratively attached along the meridians and longitudes of each doughnut hole in the cylinder according to the recipe encoded in the tree--right branch means longitude, left meridian. For our purposes the gropes will be embedded in standard form (unknotted and not self-linked) in a 3-manifold.
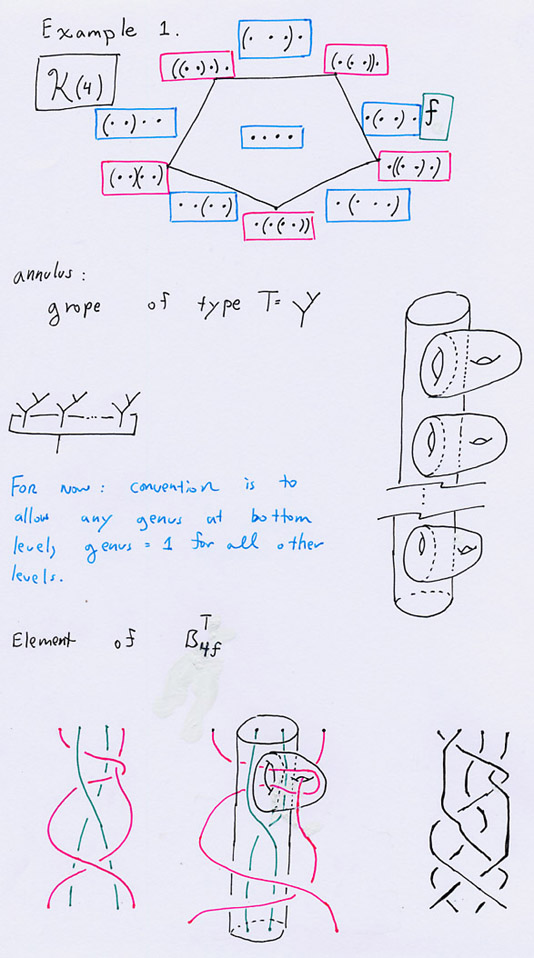
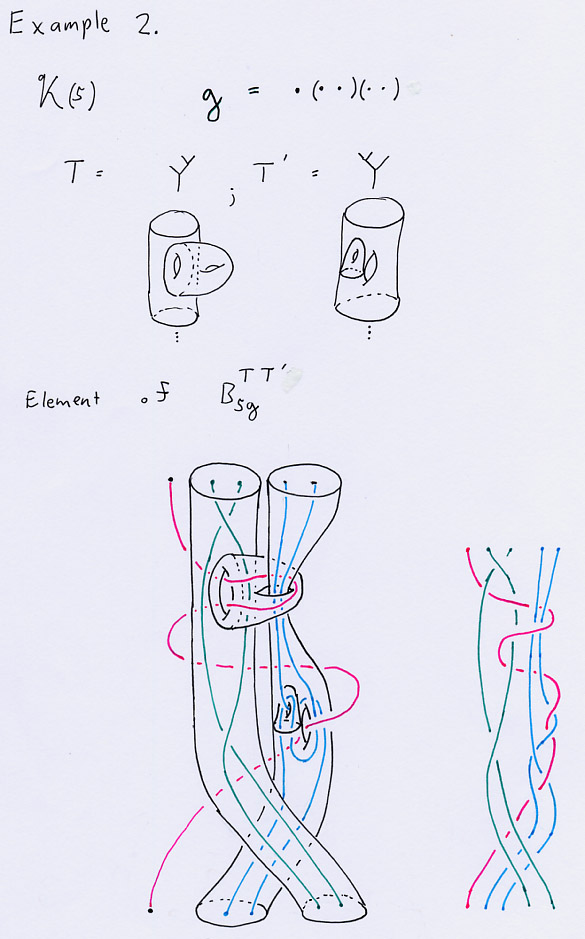
So we are considering B_n and we have chosen a facet f of K(n), the Stasheff associahedron. Furthermore, for each pair of matched parentheses in the partition of the string of length n that is associated to f, we are given a grope of type T represented by a binary tree. The constrained braids are those which allow the strands in each partition to be contained in an annular grope of the given type for that partition. In other words for a set of strands whose initial "input" points are enclosed by a pair of matched parentheses we draw a grope cylinder of the type associated to that pair of parentheses with one boundary surrounding the set of input points and the other the outputs, and require that no strands ever intersect the surface of the grope. The braid must also respect the partition associated to f by having the output points of the strands fall into the same partition, so that the braids for a given f and list of grope types form a subgroup of B_n. Examples will follow.
We wish to answer some fundamental questions about these subgroups, including finding a presentation for them, arranging filtrations involving the grope types, finding intersections of subgroups involving various combinations of associahedron facet and grope type, and relations of these subgroups to more familiar ones such as Brunnian braids and commutator subgroups. Then we investigate the link types that arise as closed elements of grope constrained subgroups. In this context we would like to find or recognize invariants that reflect the geometry of the constrained braids.

Examples:

Notice that in example two we allow intertwining of the grope constrained bundles of two strands. Thus in order to be a subgroup the strands must be allowed to be described as being constrained by mixed gropes of the two types in question. Alternatively we could simplify things by requiring that all subpartitions of a given type in the partition be assigned the same grope type. Note that in drawing the last example the strands at first appear non-monotonic, but this is just an artifact of trying to show the grope as well as the strands.

Presentations:

Given a group extension G' of a group G by a group A, by which we mean a short exact sequence

1 -> A -> G' -> G -> 1

and presentations of G and A, there is a simple recipe for a presentation of G'.

In the case of a subgroup X_n of B_n we always have the exact sequence

1 -> PX_n -> X_n -> SX_n -> 1

where PX_n is the pure version of our subgroup X_n, i.e. intersection of X_n and P_n, and SX_n is the subgroup of permutations that can be achieved as the projections of braids in X_n onto the symmetric group.

This reduces the problem of finding a presentation of a subgroup of constrained braids to finding presentations for the corresponding pure constrained braids and permutations. It seems that this problem will vary from relatively simple to quite difficult based primarily on the complexity of the grope types and secondarily on the number of strands. The hardest problems will correspond to facets of codimension n/2 in K(n). For simple cases we can proceed straightforwardly.

Here is my conjecture about the generators of the pure braids in example 1 above.

Relations anyone?

The subgroup of permutations will depend only on the facet of K(n). For the facet f = * ( * * ) * as in example 1, the permutation subgroup SB_4f^T is presented by < a, b | a^2 = b^2 = 1, ab = ba >

For another example, in K(5), consider the facet labled by ( * * )( * * * ). The permutation subgroup is presented by < a, b_1, b_2 | b_1b_2b_1 = b_2b_1b_2, a^2 = (b_i)^2 = 1, ab_i = b_ia > where i = 1,2. a is the interchange on the first two positions, b_1 switches the first two in the second group, and b_2 switches the last two.

Lots more work to be done here! We would like to start with a facet of K(n) and a list of binary trees, add labels to string elements and leaves, and then build a presentation out of those labels.

Filtrations:

Given a facet of K(n) it seems clear that there should be sequences of grope types that provide a filtration of the subgroup of B_n of braids for which the partition associated to the facet is preserved. These latter braids can be described as being "constrained" by arbitrary genus cylinders. The bottom of the filtration is given by the case in which all the grope types are trivial, that is, simply ordinary genus 0 cylinders.

Here is an example:

It will be necessary to prove a precise theorem about the sufficient complexity of a grope to be equivalent to an annuli given the number of strands or constrained groups of strands within and without it. An initial conjecture is that any grope which includes surfaces attached to both longitudes and meridians is sufficiently complex for any strand configuration. This limits the available gropes to those with slopes of 1/2 and -1/2. In these, for n outside strands, we conjecture that the height must be larger than n for the grope to be sufficiently complex.

Also, given a fixed grope type, we should be able to find a filtration of B_n based on inclusion in K(n).

Here is an example:

We would like to understand more fully the general case, and also to relate this to other filtrations of B_n such as the weight filtration and the Vassiliev filtration. There is some potential for the filtrations above to be combined into a very comprehensive family of filtrations of B_n. This is in done by beginning with a complete parenthization of the strands, corresponding to a vertex of the associahedron. Then we construct an (n-2)-dimensional array where (n-2) is the number of pairs of parentheses. The positions in the array correspond to choices of grope type for each pair of parentheses, ordered by increasing complexity. Thus the first position in the array corresponds to the trivial any genus grope for each pair of parentheses, and gives B_n itself. Moving in any direction as long as the array indices increase or remain constant means we pass from a group to one of its subgroups. Eventually by increasing the array index for any pair of parentheses the grope type will be complex enough to be considered a simple annulus for the purposes of the constrained braid. The limit in all indices then is the completely constrained braid relative to the complete parenthization--all the gropes are simple annuli.

Intersections:

Notice as in the following example that a braid is often found in two different nontrivial constrained forms.

We would like to know when to expect this sort of overlap, whether or not the intersection is a subgroup in turn, and how to achieve its presentation

Brunnian braids:

Notice how Brunnian braids are often found as constrained pure braids. Some examples:

We would like to know if this is always true. Naively it is due to the way that the constraining grope allows only "cancelling interaction" between the strands inside it and out. That is, such pairs of separated strands have relative winding numbers of 0. More broadly, this hints at a relationship with the commutatator subgroup and central series of subgroups of B_n. Here is an example of a constrained braid (from the subgroup in example 1) that lies in the second lower central subgroup of the pure braid group P_4.

This braid also exemplifies a generalization of the Brunnian braids known as the k-trivial or k-decomposable braids, in which deletion of any k strands results in a trivial braid. Specifically, deletion of any two strands in the above results in a trivial braid. Here is another 2-trivial braid that is constrained by nested gropes.

Closing:

The closure of a constrained braid is a representation of a constrained knot or link. To be a knot the partition of the strands must be "homogenous" by which we mean a partition into subsets of strands of equal cardinality. Otherwise, in order to respect the partition the braid is forced into having a closure with multiple components. Here is an example of a simple closed constrained braid that is a recognizable knot--the connected sum of a figure eight and a trefoil.

We would like to find invariants that would reflect the grope constraint. Upon inspecting a braid diagram and determining that it respects partitions (from a certain chain of included facets in the appropriate associahedron) we would like to use the diagram to calculate an invariant that could tell what grope types the braid also respects. This would be of interest especially if it afforded a new geometric understanding of existing well known invariants.

Further investigation:

1. Subcategories of the free braided category on one object: Objects of the category are partitioned strings of the one object. The strands making up a morphism would be required to obey grope constraining. The braid need not however respect the partition exactly, since we do not require that all morphisms be composable. Morphisms then exist between objects that have the same string length and congruent partitioning, such as

*(**)(**) -> (**)*(**)

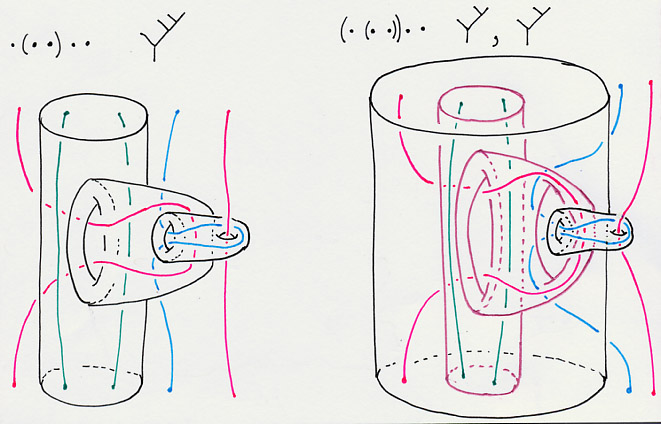
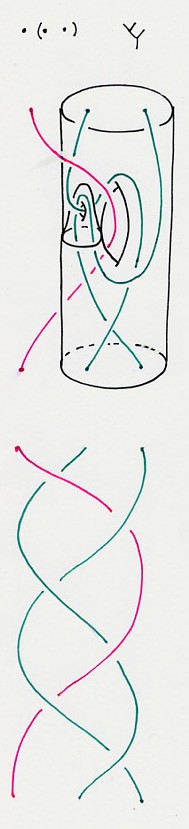
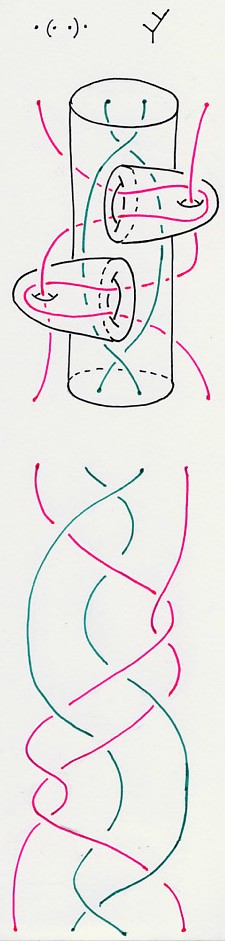
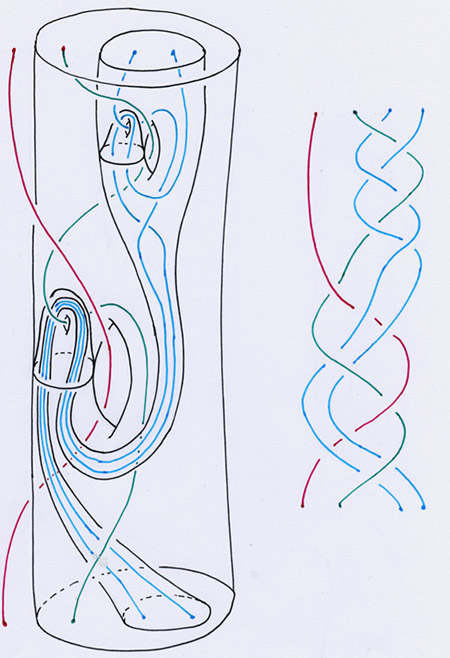
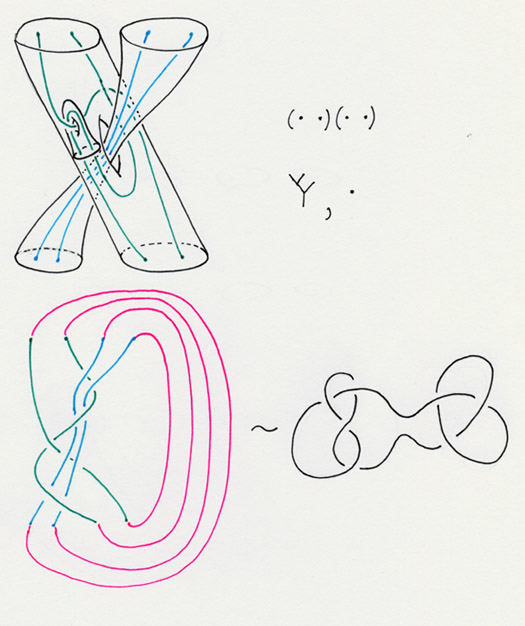
or *((***)*)(**) -> (**)(*(***))*.

2. Grope constrained string links and tangles. The string links are braids without the monotonicity requirement, and thus without existense of inverses. Tangles also allow a strand to begin and end at the same level, or to be a circle.

3. There are several intimate connections between the lower central series of pure braid groups, homotopy theory, and Vassiliev invariants. We hope to shed light on some outstanding related mysteries in part by investigating subgroups of braids constrained by gropes.

a. Fact: for two knots K1 and K2 the following are equivalent:

(i) They have the same finite type invariants of Vassiliev degree < n

(ii) They are cobordant by a grope of class n

(iii) K1 = the closure of a braid b and K2 = the closure of pb, where p is in LCS_n(P_k)

Now since P_k is the fundamental group of Config(D^2, k), p is thus the boundary of the image of a grope of class n mapped continuously into Config(D^2,k).

Question: whether there is any direct relation between the two gropes of class n that occur above? Of course there may be more than two in question since both theorems state existence--but it would be neat if the same grope was shown to occur in both contexts. It appears that any braid p in LCS_n(P_k) occurs in one or more grope constrained subgroups. Perhaps this latter grope can be compared to the others.

Plan: show the relation between the filtrations given by grope constraints and the lower central series. Especially relate the grope constrained groups to the special subgroups in the descending series, Brunnian braids and the more general k-trivial braids. Then look for canonical cobordisms and continuous maps respectively using and from the constraining gropes in question.

b. Facts: for n>3 the pure braid groups over S^2, with face maps deletion of strands and degeneracies doubling of strands form a simplicial group with geometric completion of the homotopy type of S^2. There is a surjection from the Brunnian braids on n strands to the nth homotopy group of S^2. The nth homotopy group of S^3 is given by a quotient of the Brunnian braids on n strands over S^2. The Brunnian braids are cycles in the simplicial group, the subgroup we divide by is of boundaries. Brunnian braids occur as grope constrained braids.

Question: Fred Cohen asks for a natural geometric meaning of the above mentioned boundary braids.

Plan: Look among the braids constrained by nested gropes for cycles and boundaries. If this can be made to fit the framework for discovering the homotopy groups of S^3 attempt to generalize the process and look for relations between the quotients of grope constrained subgroups of braids over S^n and the homotopy groups of S^n+1.

c. Fact: a 1+1 dimensional topological quantum field theory can be given by a choice of vectors in a finite dim. vector space V for the disk, in V*V for the annulus, and in V*V*V for the pair of pants.

Question: we would like to formulate TQFT versions of finite type invariants by describing a field theory of grope cobordisms.

Plan: We need only show how to assign an invariant to a grope annulus, since a grope trinion and a grope disk can both be cut into a grope annulus and an ordinary trinion or disk repectively. We propose then to assign to a grope annulus the group cohomology of the n-strand constrained subgroup of B_n associated to the grope type.

Back to research page. Revision Date: